modular

260: Six Intersecting Pentagonal Prisms

| wonko

Now most who know me know that I am up for a challenge and when I saw this one I knew I had to give it a go:

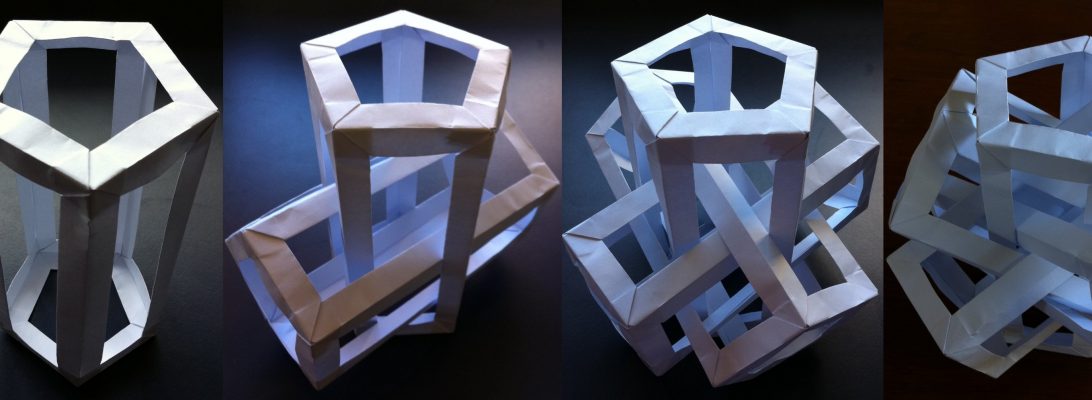
90 pieces of paper (60 small and 30 long) individually folded and locked together, no glue make an astonishing lump of awesomeness:

This has taken me AGES – folded over a the course of last week, the last two prisms were added today and we have this lovely thing. Designed by Daniel Kwan, based in part of a Francis Ow unit, the angles necessary to make a pentagon are tricksey.

The tab and pocket construction technique is, in theory, really simple but when the model has 3 simultaneous tabs (for any vertex) keeping them all in before locking them was really fiddly and resulted in much swearing. As the model got more and more crowded the problems increased to the point where I nearly gave up, having mangled a set of tabs so badly they were not going to insert, requiring a refold.

Very satisfying to finally finish – there is a lovely symmetry with this model – pentagonal swirls framed by pentagons. I think my term 3 modular is cool – hope you like it also.

Want to make it? Download my intersectingPentagularPrismsPattern and print it on an A3 page, cut out the shapes and get bending – tab A goes into slot B etc. Originally this was designed to be made from STARBURST lolly wrappers but I scaled them up to be double that to make it easier. Achievable with copy paper, probably much easier with a different colour for each prism in retrospect.

252: Umulus Rectangulum

| wonko

I liked this modular when I first saw it and knew that it would look wonderful in colour:

An interesting elbow bend, tubular construction and odd folding in fifths make this model interesting to make

You start with a split A4 page, box pleat and lock the tube, then slide one inside the other to make a rectangular hoopy thing, then nest another inside that one, then lock a third over the top of that one and you end up with a lovely sort of impossible looking shape

I like this a lot, the illusion of intersecting shapes in well designed and although a little time consuming it is satisfying and a keeper I think.

237: Fuse’s Triangle Box

| wonko

Tomoko Fuse is renowned for her intricate boxes – this one is a version of her triangle box:

A modular, top and bottom each made up of 3 modules that interlock and lace together to create a curious container

I must explore these modular boxes some more, when I have more time, quite happy with this as my first fold however.

222: Jitterbug

| wonko

My Monthly Modular is a fab use of equilateral triangles that work in clusters of 4 or 5 to make interesting spheroids:

Eight of the modules make a “Jitterbug” – a curious structure that twists into a cube.

Thirty of the modules clustered in fives make a soccer ball (er, sorry, an Icosadodecahedron) which is a lovely thing to behold. (oops, sorry, a icosidodecahedron)

A relatively simple construction, quite quick also and also fairly rigid given how the parts interlock and self-tighten. You can have a go at this yourself here

202: Ninja Star

| wonko

When I was a kid, an old black and white telly series from Japan called “Samurai” captured my attention – corny stories of good guys in white versus ninjas in black:

They threw star knives as one of their many skills (jumping, film reverse, back up into corners roof corners was another). A year 6 student brought me a ninja star he had made for him (mum or dad folded it) after I inquired if he could teach me.

After a small amount of deconstruction, the elegantly simple construction was evident – strength and simplicity in what looks like an intricate machine. I liked it so much I made a coloured one, using some lovely little washi squares Mary bought for me (love your work Cass’)

Happy with this, particularly as the star moves upon itself and the “blades” retract into a lovely octagonal ring – very clever

Tetrahedra Revisited

| wonko

…so I was bugged that as tiny little triangles, in white, I found it impossible to complete the 5 intersecting shape thing, so I went to the school copy room and asked for some colourful Copy paper – A4.

I got 5 strong colours, cut squares, made them into thirds, total of 6 strips per tetrahedra, 5 tetrahedra – total of 30 bits of paper, 1-2 minutes to fold each unit, 3-5 minutes each to place and lock into surrounding units and it is done.

I find this shape fascinating, and the order of the pattern was only evident after I had completed it – from simple shapes, great complexity and beauty can arise.

142: Interlaced Tetrahedra

| admin

Now I have never tried MODULAR origami – it is a huge and enthusiastic movement in the society of paper benders – making modules that lock together to make a compound shape. I found instructions for Francis Ow’s 60° Unit and thought I would give a tetrahedron (6 of these units) a go:

I … got a little carried away and discovered they can nestle amongst each other in a lovely geometrically interesting sort of way. With this module, apparentyl, it is possible to complete 5 (yes, you heard me, 5 = 5×6 = 30 strips of paper) intersecting tetrahedra – scale beat me here (the tiny units are just too fiddly to lock together – must try it on A4 scale).

This is a new frontier for me, and it interests me strangely – the units are self-centring, lock each other so require no glue, have an amazing tensile strength when locked together and are simple to fold (1-2mins each). Based on 1/3 of a square, the folded thickness apparently is mathematically proved to allow a 5-intersection – we shall see.

You should try this – I enjoyed the modular approach and will probably try another in the next month (there are lots of flavours that do all sorts of things geometrically speaking) – Engineers (and those budding ones) should have a fiddle – something stunningly beautiful about regular geometry (better not say this too loud, maths teachers might hear me)